Riemannsche Geometrie (Riemannian Geometry)
News
- The course will be an online-course. Please register in Moodle (all material will be provided there!).
Prerequisites
This course is aimed at students with interest in analysis and geometric objects.
The lectures may - and will - be adapted to the individual needs and interests of its participants. In case you have any suggestions for the content or the feeling that relevant topics need to be repeated, we kindly ask you to get in touch with the course instructors.
Course Content
Riemannian geometry is the intrinsic study of manifolds with a metric, so-called Riemannian manifolds. Here intrinsic means that the quantities we define have to be observable by 'inhabitants' of the manifold.
The approach differs from the classical differential geometry in the way that we do not look at surfaces from outside by choosing parametrizations, but rather we put ourselves into the position of an inhabitant of a manifold who just knows the manifold and nothing else. We study whether and how such an inhabitant can find shortest paths between two points, so-called geodesics.
The Riemannian metric determines how an inhabitant can measure velocities and lengths. The problem is that the metric may vary heavily throughout the manifold. This is - essentially - also the reason why time runs slower close to a black hole.
We investigate how inhabitants can detect curvature of the manifold (if they can at all do that). This matches up with an everyday experience: Even though we know that the earth's surface is a sphere, we are unable to see with our eyes that it is curved. So how can we really prove that it is curved?
The field was initiated by Riemann 1854 in his Habilitation lecture "Über die Hypothesen, die der Geometrie zugrunde liegen". We quote a very central result from his lecture manuscript:
"Der gemeinsame Charakter [von] Mannigfaltigkeiten, deren Krümmungsmass constant ist, [ist], dass sich die Figuren in ihnen ohne Dehnung bewegen lassen." (A common property of manifolds of constant curvature is, that one can move objects in them without stretching)
Proving that objects are curved also becomes important when looking at general relativity, more exactly when looking at the (curved) spacetime we live in. In this setting however the metric does not really fit into Riemann's original assumptions. Conceptionwise however many things carry over.
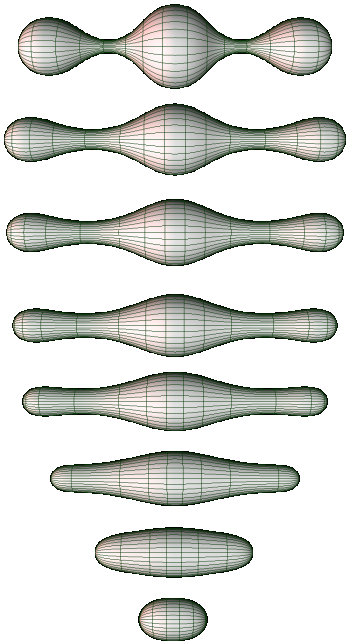
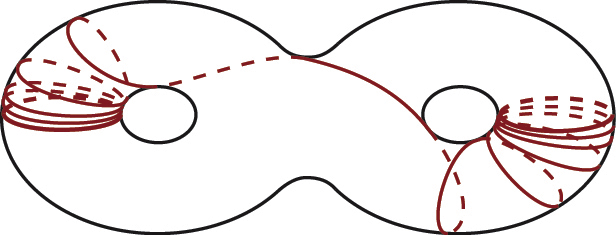
Riemannian geometry is still important nowadays in mathematics. The only solved ''Millennium Problem'' of the Clay Mathematics Institute is the so-called Poincaré conjecture. It was solved in 2002 by G. Perelman using the Ricci Flow (see video), which is an ongoing and vivid field of research.
Admission to the Exam
In order to be able to take the exam, 50% of the Moodle Mini-Quiz points are required. Please check our moodle page for details.
The exam will be an oral exam.
Literature
Bär, Christian(D-PTDM-IM)
Elementare Differentialgeometrie. (German) [Elementary differential geometry]
Walter de Gruyter & Co., Berlin, 2010. xvi+340 pp. ISBN: 978-3-11-022458-0
do Carmo, Manfredo P.(BR-IMPA)
Differential geometry of curves & surfaces.
Dover Publications, Inc., Mineola, NY, 2016. xvi+510 pp. ISBN: 978-0-486-80699-0; 0-486-80699-5
do Carmo, Manfredo Perdigão(BR-IMPA)
Riemannian geometry.
Mathematics: Theory & Applications. Birkhäuser Boston, Inc., Boston, MA, 1992. xiv+300 pp. ISBN: 0-8176-3490-8
Jost, Jürgen(D-MPI-NS)
Riemannian geometry and geometric analysis.
Universitext. Springer, Cham, 2017. xiv+697 pp. ISBN: 978-3-319-61859-3; 978-3-319-61860-9
Jost, Jürgen(D-BCHM-MI)
Differentialgeometrie und Minimalflächen. (German) [Differential geometry and minimal surfaces]
Springer-Lehrbuch. [Springer Textbook] Springer-Verlag, Berlin, 1994. xii+151 pp. ISBN: 3-540-56904-9
Morgan, Frank(1-WLMS)
Riemannian geometry. A beginner's guide. A K Peters, Ltd., Wellesley, MA, 1998. x+156 pp. ISBN: 1-56881-073-3
Further literature can be found in the library in the bookshelf "Semesterapparate".
Lecture Time and Place
Lectures:
- Tuesday, 14-16 h, Helmholtzstrasse 18, E20
- Thursday, 12-14 h, Helmholtzstrasse 22, E04
Exercise Classes:
- Monday, 14-16 h, Helmholtzstrasse 18, 120